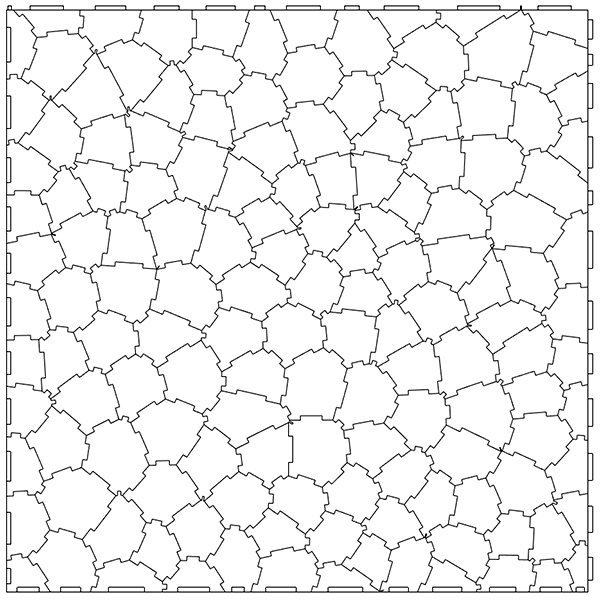
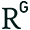Voronoi Kündekâri

Kündekâri is an old woodworking technique, composed of interlocking parts without any glue or nail. It is primarily used in wooden doors and minbers inside Mosques. The interlocking system makes the whole structure very durable. I tried to model this technique using the Voronoi pattern, naming it Voronoi Kündekâri. Below you see a typical example of Kündekâri pieces and the resulting pattern.

The interesting part of the Grasshopper model is to define the tongue-and-groove detail. It is similar to a puzzle. Although it is very easy in Rhino to create it, I found it challenging to implement this detail with a non-regular subdivision such as Voronoi. In a regular subdivision such as hexagons, it was way much easier, such as the Trifoliolate (explained here). You have to tell Grasshopper which edge is tongue and which edge is groove one by one. In the real world, it is a very simple decision, but it is a mess for a dataflow algorithm. Because you should consider a random Voronoi subdivision, with cells composed of 3, 4, 5, or 6 edges. So, as you can see in the diagram below, I tried many tricks to solve this problem.

You can click on the diagram to see it larger. My process was simply splitting the edges, offsetting, and connecting the ends.

You can rebuild the definition by looking at the definition above. However, if you want to support this website by downloading my Grasshopper file; would you consider being my Patreon? Here is the link to my Patreon page including the working Grasshopper files for the Voronoi Kündekâri and more.